4. Domain Theory

A remarkable property of ferrimagnetic materials is not so much that they have a spontaneous magnetization, but rather that their magnetization can be influenced by the application of very low magnetic fields. Even the earth's field (50 μT) can cause magnetization changes even though the interatomic exchange forces responsible for the spontaneous magnetization are equivalent to a field of about 1000 T, almost 100 million times greater than the earth's field.

What allows this to occur is the fact that the sample is actually composed of small regions called magnetic domains, within each of which the local magnetization is saturated but not necessarily parallel. Domains are small (1-100's microns), but much larger than atomic distances.

The existence of domains is hinted at by the observation that some magnetic properties, and in particular, coercivity and remanence vary greatly with grain size. This is best illustrated in the figure below, which shows the variation of H_c with grain size.

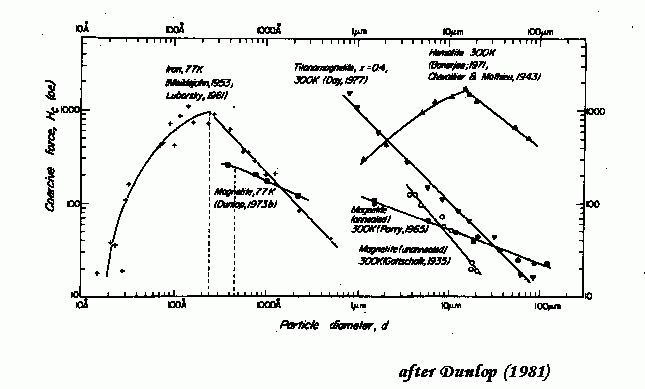
The magnetic behavior can be subdivided on the basis of grain size into four ranges

1. SPM: superparamagnetic
2. SD: single domain
3. PSD: pseudo-single domain
4. MD: multidomain

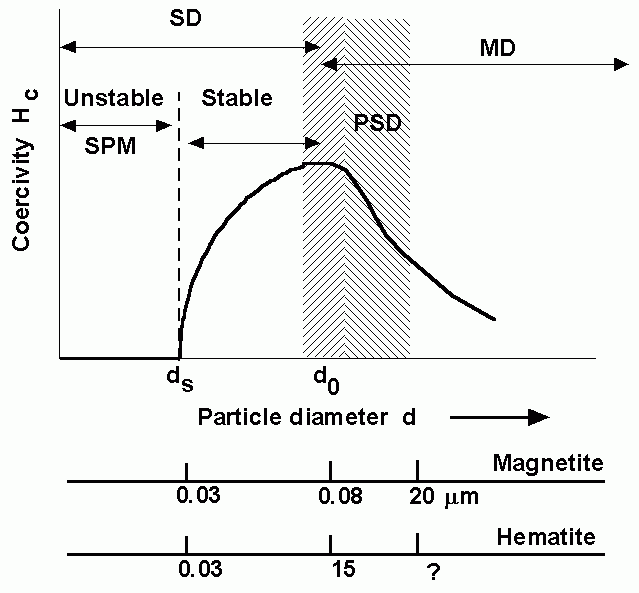
The maximum coercivity for a given material occurs within its SD range. For larger grain sizes, coercivity decreases as the grain subdivides into domains. For smaller grain sizes, coercivity again decreases, but this time due to the randomizing effects of thermal energy.

Domains constitute a fundamental concept in magnetism. A ferro- or ferrimagnetic material may be generally defined as one that possesses a spontaneous magnetization, Ms, dependent on temperature, but only slightly dependent on applied field. The theory of ferromagnetism, based on electronic exchange forces, predicts the magnitude of Ms, but says nothing about the direction of Ms. Experimentally, it is observed that for a homogeneous specimen at constant temperature, the magnitude of Ms is uniform but the direction of Ms is in general not uniform from one region to another (on a scale of microns to millimeters). Uniformity of direction is attained only by applying a large enough field to drive the domains out of the sample, or by reducing the particle's dimensions to small enough size to prevent domain formation.

Domains are formed for the following reason. Consider a large single crystal.

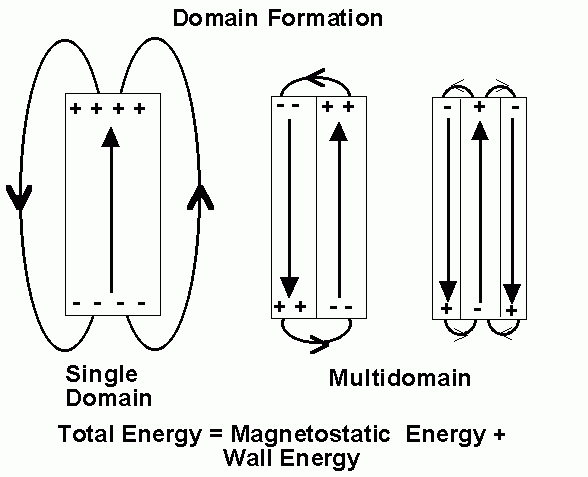
Suppose it is uniformly magnetized, and hence a single domain. Surface charges will form on the ends due to the magnetization and are themselves a second source of a magnetic field (the demagnetizing field). The energy associated with the surface charge distribution is called the magnetostatic energy. It is just the volume integral of the field over all space.

The magnetostatic energy can be approximately halved if the magnetization splits into two domains magnetized in opposite directions. This brings (+) and (-) charges closer together, thus decreasing the spatial extent of the demagnetizing field.

This subdivision into more and more domains can not continue indefinitely because the transition region between domains (called a domain wall) requires energy to be produced and maintained. Eventually an equilibrium number of domains will be reached for a given particle size.

Domain walls are interfaces between regions in which the magnetization has different directions. Within the wall, the magnetization must change direction from that in one domain to that in the other domain. Domain walls have a finite width that is determined principally by exchange and magnetocrystalline energy.

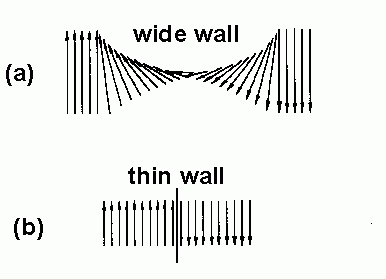
Let's consider a domain wall in which the magnetization changes by 180°. The change in magnetization within the wall can be gradual as in (a) or abrupt as in (b).

The exchange energy acts to keep spins parallel and can be kept small if the 180°rotation takes place gradually, over many atomic units. Therefore, the exchange energy is small in (a) but large in (b).

However, the spins within the wall are no longer aligned along an easy axis of magnetization. This produces an anisotropy energy, which is high in (a) but low in (b).

The exchange energy tends to make the wall as wide as possible whereas the anisotropy tends to make the wall as thin as possible. As a result of this competition between exchange and anisotropy energies,the domain wall has a finite width (on the order of 100 nm) and surface energy.

The interplay between long range and short range effects results in the domain states being grain-size dependent. In addition, the number of domains for a given grain size depends on the magnitudes of the exchange, magnetocrystalline, and saturation magnetization. As mentioned before, these constants are dependent on temperature as well as composition. Hence domain states in different magnetic minerals (magnetite and hematite) will have a different grain size dependence. The domain states will also vary with temperature for a single grain size. However, as a rule of thumb, the larger the grain size the more domains it contains.

 

Single Domain (SD)

As the grain size decreases, a critical size will be reached where the grain can no longer accommodate a wall. Below this critical size, the grain contains a single domain (SD). An SD grain is uniformly magnetized to its saturation magnetization.

SD grains are very important. To change the magnetization of a MD grain, all you need to do is translate the domain wall, a energetically easy process, which can be accomplished in relatively low fields. Thus MD grains are magnetically soft with low values of coercivities and remanence.

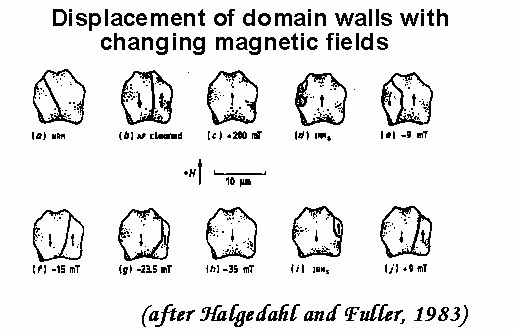
However, the only way to change the magnetization of a SD grain is to rotate the magnetization, an energetically difficult process. Thus, SD grains are magnetically hard and have high coercivities and remanence. Here is an example of an SD and MD grain as characterized by hysteresis loops:

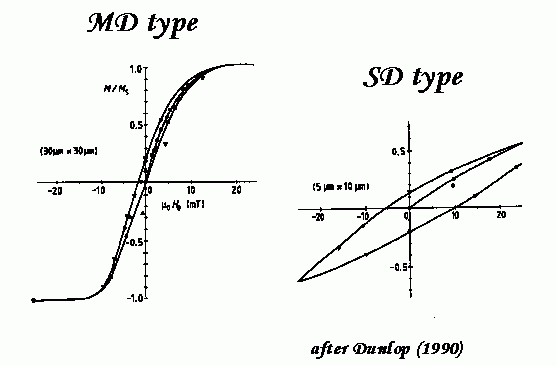
The critical size for SD behavior depends on several factors including, the saturation magnetization and the shape of the grain. Most estimates of the SD-MD transition size are based on theoretical calculations. For magnetite, the best estimate for the transition size is about 80 nm. Here are some theoretical results: 

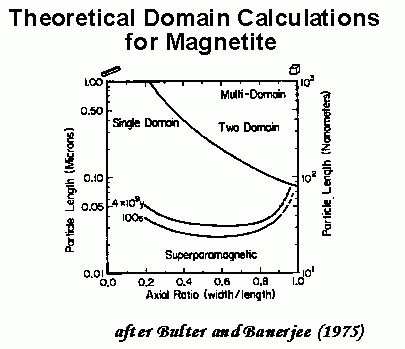
For hematite, the transition size from SD to MD is much larger (15 mm), primarily because the saturation magnetization is about 200 times lower than for magnetite.

 

Pseudo-Single Domain (PSD)

The distinction between SD and MD is straightforward. However, small MD grains exhibit a mixture of SD-like (high remanence) and MD-like (low coercivity) behavior. For magnetite, this behavior occurs in the size range between 0.1-20 μm.

There has been much theoretical and experimentally work on PSD grains. Some current thinking is that small MD particles that contain just a few domains may actually have difficulty nucleating domains. In some cases MD grains exist in metastable SD states. The transformation of one domain state into another, such as addition or loss of domains, is call transdomain transformation.

The importance of PSD behavior in magnetite, is that the grain size range for PSD behavior covers the range in sizes that most commonly occur in natural samples.

 

Superparamagnetism (SPM)

As particle size continues to decrease within the SD range, another critical threshold is reached, at which remanence and coercivity go to zero. When this happens, the grain becomes superparamagnetic.

An SD particle of volume v has a uniform magnetization directed along the easy axis of magnetization. If v is small enough, or the temperature is high enough, thermal energy (kT) will be sufficient to overcome the anisotropy energy separating the (+) and (-) magnetization states and cause a spontaneous reversal of magnetization.

For superparamagnetic particles, the net magnetic moment in zero field and at T >0K, will average to zero. In an applied field, there will be a net statistical alignment of magnetic moments. This is analogous to paramagnetism, except now the magnetic moment is not that of a single atom, but to an SD particle containing 10⁵ atoms. Hence, the term superparamagnetism, which denotes a much higher susceptibility value than that for simple paramagnetism.

In response to a change in the applied field or temperature, an ensemble of SPM particles will approach an equilibrium value of magnetization with a characteristic relaxation time, first derived by Néel:

where

f₀ -frequency factor (10⁹sec^-1)

K_u -anisotropy constant

v -particle volume

k -Boltzmann constant

T -absolute temperature

The exponential nature of the relaxation time on v and T makes it possible to define a blocking temperature, T_B (at constant volume), or blocking volume v_B, (at constant temperature) at which the magnetization goes from an unstable condition (t<<t) to a stable condition (t>>t). For example,

	t (sec)
21	1
60	1017

In spherically shaped magnetite at room-temperature, this change in energy corresponds to an increase in size from only 22 to 33 nm!

In nature, fine particles of magnetite can acquire stable remanence as they pass through the blocking conditions as they cool from high temperatures or grow authigenically or diagenically at low temperatures.

 

Initial Susceptibility

Initial susceptibility is measured in a low AC or DC field (<1mT) and is defined as the ratio of M/H. Initial susceptibility is due to reversible displacements of mobile domain walls in MD particles or moment rotation in SD particles. In the latter case, low fields are not very effective in rotating SD moments. Therefore, susceptibilities in SD and PSD grains are usually lower than that of MD grains.

However, what is actually measured in the laboratory is the apparent susceptibility, χ_o, not the intrinsic susceptibility, χ_i. The difference is due to the effects of self-demagnetization.

Remember, inside a grain, the applied field, H, is modified by the demagnetizing field resulting from surface charges. The magnitude of the demagnetizing field is NM. Inside a grain, the internal field is

H_i = H-NM

M =χ_iH_i

The observed susceptibility is the ratio of M to the applied field

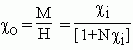
For strongly magnetic materials, like magnetite

Nχ_i > 1

χ_o » 1/N

N is weakly related to grain shape and domain state. It is usually assumed to be a constant, independent of grain size. If this is so, low-field susceptibility can be used as a reliable measure of magnetite content.

A small fraction of SPM particles can contribute significantly to the room-temperature susceptibility of SD or MD grains. Calculations show SPM susceptibility can be 10-100 times that of an equivalent amount of SD grains.

 

Frequency Dependence of Susceptibility

Low-field susceptibility can also be measured at different frequencies of the applied AC field. SPM grains show the most pronounced frequency dependence of low-field susceptibility. Changing the measurement frequency is basically changing the amount of time allowed for the grains to react to a change in applied field. This is the same as changing the blocking volumes. As the frequency of the measurement increases, the SPM/SD boundary shifts to smaller volumes and more grains become blocked.

Experimental results show that the % decrease in χ_o per decade of frequency is:

1-20%	SPM grains
<1%	SD,MD grains

 

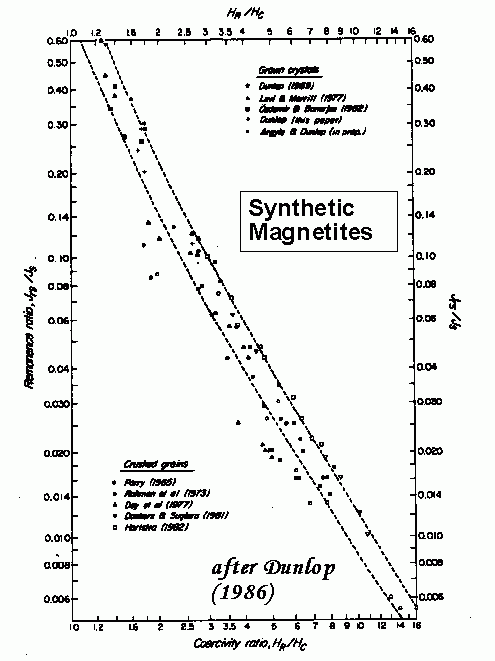
Hysteresis Properties of SD, PSD, and MD Particles

The shape of a hysteresis loop is determined partly by the domain state. Loops for SD materials are typically wider than loops for MD materials. This is just a reflection of the higher coercivity and remanence in SD material. The hysteresis loop parameters, Mr/Ms and Hr/Hc>, have proven very useful in distinguishing domain state. In fact, Mr/Ms is a definitive test for differentiating between SD and non-SD particles. Here are results for magnetite:

 

SD Hysteresis Properties

For an assembly of SD grains with randomly oriented easy axes, Mr/Ms can be calculated and depends on the type of anisotropy:

Type of Anisotropy	Mr/Ms	Source
uniaxial	0.5	shape,stress
magnetocrystalline (cubic)
K1 < 0	0.87	intrinsic
K1 > 0	0.83	intrinsic

Hc > 10-15 mT for equidimensional particles

Hc > 30-40 mT for acicular particles

Hr/Hc = 1-2

 

MD and PSD Hysteresis Properties

For an assembly of MD or PSD particles, it is more difficult to calculate Mr/Ms and Hr/Hc ratios. From models based on displacements of mobile domain walls and experimental results on synthetic samples, the following values are typical:

Parameter	PSD	MD
Mr/Ms	0.1-0.5	<0.1
Hr/Hc	2-4	>4
Hc	10-15 mT	<10 mT

 

SPM Hysteresis Properties

SPM particles exhibit no remanence or coercivity. The shape of the hysteresis loop is thus extremely thin. SPM grains show a very steep initial rise in magnetization with field and then a more gradual increase to saturation. However, in a mixture of mostly SPM grains but with some SD or MD grains, typical values for hysteresis parameters are:

Mr/Ms << 0.01

Hr/Hc > 10

Separation of SPM from MD grains based on hysteresis properties can be a problem using just room temperature measurements. In some cases, cooling the sample down to very low temperatures can be helpful.

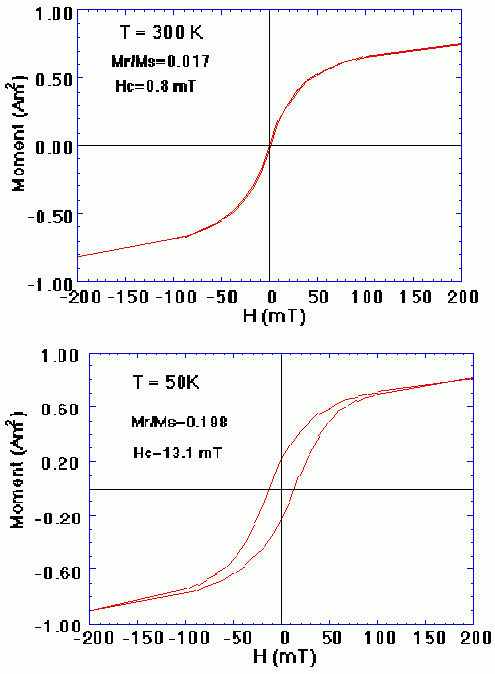
Grains that are SPM at room temperature can become blocked and SD at low temperatures. Significant changes in hysteresis parameters between room temperature and 77K is diagnostic of a SPM contribution.

 

Summary

As shown above, the Mr/Ms -Hr/Hc diagram is a useful indicator of domain states

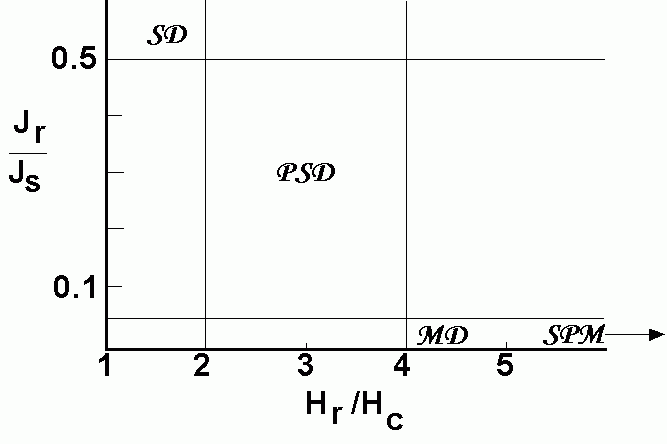
Mixtures of Domain States

Often, natural samples may contain two populations of grain sizes, a coarse MD fraction and a fine SD fraction. This makes the interpretation of hysteresis data more complicated. For example, Hr/Hc is biased toward the low-coercivity fraction, as shown here

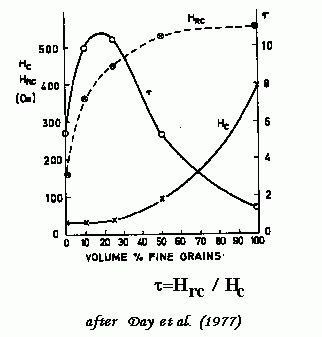
Very high values of this ratio indicates SPM particles.